nLab Shum's theorem

Statement

Proposition

(Shum's theorem)
The category of framed oriented tangles $FrOrTang$ is equivalently the free ribbon category generated by a single object.

(Shum 1994, Yetter 2001 Thm. 9.1)

The following table indicates how the main axioms for ribbon categories translate to the corresponding moves on (framed, oriented) tangle/link diagrams (cf. e.g. Selinger 2011, Baez & Stay 2011), namely to isotopy moves and Reidemeister moves:

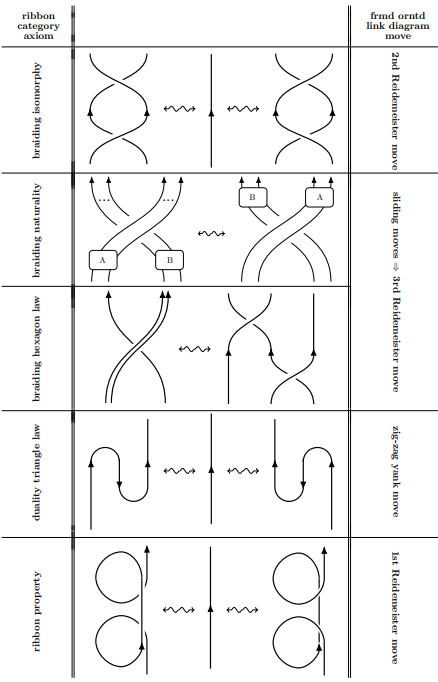
(NB: For the sliding moves, the corresponding mirrored moves are not shown, to save space.)

Relation to knot invariants

This means that for $\mathcal{R}$ any ribbon category and $V \in \mathcal{R}$ any object, there is a unique ribbon functor

(1)$$I^{\mathcal{R}}_V \;\colon\; FrTang \longrightarrow \mathcal{R}$$

which sends the generating object $1 \in FrTan$ to $V \in \mathcal{R}$.

Since the endomorphisms of the object $0 \in FrOrTang$ are the oriented framed links this means that the functor $I^{\mathcal{R}}_V$ (1) restricts on endomorphisms to a collection of link invariants (with values in the endomorphism ring of $V$ whenever $\mathcal{R}$ is Ab-enriched).

The standard link invariants arise this way (e.g. the HOMFLY polynomial), with the ribbon category $\mathcal{R}$ being the category of representations (finite dimensional) of a quantum group (cf. Yetter 2001 p 118).

References

• Mei Chee Shum, Tortile tensor categories, Journal of Pure and Applied Algebra 93 1 (1994) 57-110 [10.1016/0022-4049(92)00039-T]

• David N. Yetter: Functorial Knot Theory – Categories of Tangles, Coherence, Categorical Deformations, and Topological Invariants, Series on Knots and Everything 26, World Scientific (2001) [doi:10.1142/4542]